Dedicated Electronic Flight Computer | Aircraft Weight and Balance

Figure 1. Dedicated electronic flight computers are programmed to solve weight and balance problems, as well as flight problems

Figure 2. Weight and balance data of a typical nosewheel airplane

The problem just solved with an electronic calculator and an E6-B can also be solved with a dedicated flight computer using the information shown in Figure 2. Each flight computer handles the problems in a slightly different way, but all are programmed with prompts that solicit the required data to be inputted so there is no need to memorize any formulas. Weight and arms are inputted as called for, and a running total of the weight, moment, and CG are displayed.

Typical Weight and Balance Problems

A hand-held electronic calculator like the one in Figure 1 is a valuable tool for solving weight and balance problems. It can be used for a variety of problems and has a high degree of accuracy. The examples given here are solved with a calculator using only the (×),(÷),(+),( – ), and (+/–) functions. If other functions are available on your calculator, some of the steps may be simplified

Determining CG in Inches From the Datum

This type of problem is solved by firs determining the location of the CG in inches from the main wheel weighing points, then measuring this location in inches from the datum. There are four types of problems involving the location of the CG relative to the datum.

Nosewheel Airplane With Datum Ahead of the Main Wheels

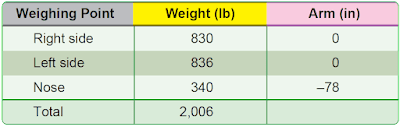
The datum (D) is 128 inches ahead of the main wheel weighing points; the weight of the nosewheel (F) is 340 pounds, and the distance between main wheels and nosewheel (L) is 78 inches. The total weight (W) of the airplane is 2,006 pounds. Refer to Figure 3.

Figure 3. Locating the CG of an airplane relative to the datum

1. Determine the CG in inches from the main wheel:
(340)(×)(78)(÷)(2006)(=) 13.2

Nosewheel Airplane With Datum Behind the Main Wheels

The datum (D) is 75 inches behind the main wheel weighing points, the weight of the nosewheel (F) is 340 pounds, and the distance between main wheels and nosewheel (L) is 78 inches. The total weight (W) of the airplane is 2,006 pounds. Refer to Figure 4.

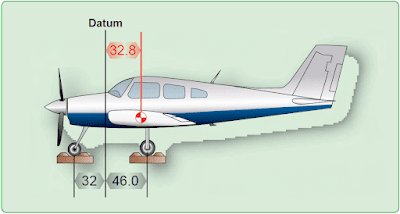Figure 4. The datum is located at the firewall

1. Determine the CG in inches from the main wheels:
(340)(×)(78)(÷)(2006)(=) 13.2

Tailwheel Airplane With Datum Ahead of the Main Wheels

The datum (D) is 7.5 inches ahead of the main wheel weighing points, the weight of the tailwheel (R) is 67 pounds, and the distance between main wheels and tailwheel (L) is 222 inches. The total weight (W) of the airplane is 1,218 pounds. Refer to Figure 5.

Figure 5. Determining the CG

1. Determine the CG in inches from the main wheels.
(67)(×)(222)(÷)(1218)(=) 12.2

Tailwheel Airplane With Datum Behind the Main Wheels

The datum (D) is 80 inches behind the main wheel weighing points, the weight of the tailwheel (R) is 67 pounds, and the distance between main wheels and tailwheel (L) is 222 inches. The total weight (W) of the airplane is 1,218 pounds. Refer to Figure 6.

Figure 6. The datum is 100 inches forward of the wing root leading edge

1.Determine the CG in inches from the main wheels:
(67)(×)(222)(÷)(1218)(=) 12.2
2.Determine the CG in inches from the datum:
(80)(+/–)(+)(12.2)(=) –67.8
The CG is 67.8 inches ahead of the datum.

Determining CG, Given Weights, and Arms

Some weight and balance problems involve weights and arms to determine the moments. Divide the total moment by the total weight to determine the CG. Figure 7 contains the specifications for determining the CG using weights and arms.

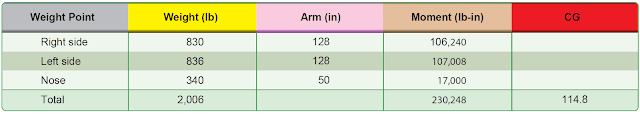Figure 7. Specifications for determining the CG of an airplane using weight and arm

Determine the CG by using the data in Figure 7 and following these steps:

1. Determine the total weight and record this number:
(830)(+)(836)(+)(340)(=) 2,006

Determining CG, Given Weights, and Moment Indexes

Other weight and balance problems involve weights and moment indexes, such as moment/100 or moment/1,000. To determine the CG, add all the weights and all the moment indexes. Then, divide the total moment index by the total weight and multiply the answer by the reduction factor. Figure 8 contains the specifications for determining the CG using weights and moments indexes.

Figure 8. Specifications for determining the CG of an airplane using weights and moment indexes

Determine the CG by using the data in Figure 8 and following these steps:

1. Determine the total weight and record this number:
(830)(+)(836)(+)(340)(=) 2,006

Determining CG in Percent Mean Aerodynamic Chord (MAC)

• The loaded CG is 42.47 inches aft of the datum.
• MAC is 61.6 inches long.
• LEMAC is at station 20.1.

Determining Lateral CG of a Helicopter

For a helicopter, it is often necessary to determine not only the longitudinal CG, but the lateral CG as well. Lateral CG is measured from butt line zero (BL 0). All items and moments to the left of BL 0 are negative, and all those to the right of BL 0 are positive. Figure 9 contains the specifications for determining the lateral CG of a typical helicopter.

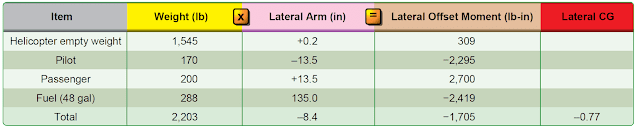Figure 9. Specifications for determining the lateral CG of a helicopter

Determine the lateral CG by using the data in Figure 9 and following these steps:

1. Add all of the weights:
(1545)(+)(170)(+)(200)(+)(288)(=) 2,203

Determining ΔCG Caused by Shifting Weights

Fifty pounds of baggage is shifted from the aft baggage compartment at station 246 to the forward compartment at station 118. The total airplane weight is 4,709 pounds. How much does the CG shift?

1. Determine the number of inches the baggage is shifted:
(246)(–)(118)(=) 128

(50)(×)(128)(÷)(4709)(=) 1.36

Determining Weight Shifted to Cause Specified ΔCG

How much weight must be shifted from the aft baggage compartment at station 246 to the forward compartment at station 118 to move the CG forward 2 inches? The total weight of the airplane is 4,709 pounds.

1. Determine the number of inches the baggage is shifted: